Characteristics of the Economic Repair Time of the Components in Public Rental Housing
ⓒ Copyright Korea Institute of Ecological Architecture and Environment
Abstract
Building has required the repair money to improve or maintain the decent living condition continuously after construction. It needs to grasp the building deterioration to decide the scope and contents before it is repaired. Under various conditions such as physical, social and financial constraints, the repair plan would be prepared. Among constraints, the cost is indispensible to specify the repair time, repair scope and target. The required cost would be planned to preparation over the years.
In this paper, it aimed at providing the repair strategy of the public rental housing in repair time, using the cumulative cost model which is 3rd function. In the 3rd function, the inflection point should exist in the line. And there are two types in the cumulative model, First, if the maximum cost be shown, the repair time would be provided. Second, if the maximum cost not be shown, the cumulative function should be proportionally increased and the repair strategy is properly provided with a short cycle. In results of this study, 11 items would provide the repair time. These cumulative function would be repaired about 4 years after constructed, and after about 4 years, the cumulative function would be continuously increased.
Keywords:
rental housing, economic, repair time, components, materials, repair model1. Introduction
1.1. Background and Purpose of the Study
Housing is supplied in two major modes, which are, respectively, sales and leases. Houses that are sold to the new owners are supplied in a mode that is focused on profits, while leasing is more concerned on supplying the spaces for living and mainly initiated by the public sector. Mainly the local authorities, or national governments, or public agencies build these houses and provide them in a lease agreement to the households that require living spaces, usually at lower rents.
This has been planing the role of the means to provide housing to the low income classes. However, the residences and the management thereof are separated. That is, as the tenants, who are not owners of those facilities, live in those houses, sometimes there are problems occurring in terms of the use or maintenance of the buildings. In addition, while the tenants live in multi-unit houses, they tend to show a lower level of awareness on the self-autonomous management of the facilities compared to the home owners. For this reason, the wear and tear of the complex continues, resulting negative impressions on those houses. For this, it is necessary to establish maintenance data bases on the parts and materials used in leased public housings, so that a management entity may systematically and continuously manage such houses.
The types of information required to manage leased houses include the repair costs after completion of the building over time. Therefore, the managing entities take steps, including depositing reserves for remodelling or repair costs in order to ensure continued management of the materials or parts. For this, it is important to understand the characteristics of the maintenance cost of the materials or the type of repair works involved.
The maintenance works, including repairs, should reflect various degree of wear and tear of the facility. At the same time, such works should be performed within a reasonable amount of budget at a reasonable time. For this, it is necessary to identify an appropriate level of such works, so that the performance of the facility can be maintained. Likewise, it is necessary to establish a strategy for maintenance, by determining the right time for maintenance works through the relationship with the costs for remodelling or repair works. That is, it is necessary to set the time of repair work in consideration of the maintenance cost involved and the limited maintenance budget. The purpose of this study is to suggest an economic repair time by analyzing the relationship between the required cost for remodelling or repair works and the elapse of time. As such, the result of this study can be used as an important input for establishing maintenance plans for public rental houses after their completion, covering the repair costs and the time of repair works for materials or components. In addition. the result of this study may be used in estimating the approximate amount of repair costs based on the frequency of repair works for the materials.
1.2. Contents and Methods of the Study
Leased public housing is a type of housing supply aimed to provide living spaces to the low income class of the society. The supplying entity in this case is the local authority, the national government, or a public agency, which are also the entities responsible for managing of the facilities. The managing entity tend to perform economic maintenance, with limited amounts of budges to maintain the performance or functions of lease public houses. The purpose of this study is to suggest economic repair times of the components of public rental housing. The method of study used to fulfill this goal is as follows;
Firstly, cubic functions were used as the model to analyse the economic repair characteristics of public rental houses. By using this type of functions, the relationship between the age in years and the repair cost was analyzed. Here, the model to explain the repair cost and the age in years used the statistical values to analyze the significance of the analysis model. Using cubic functions, the relationship between the ages in years and the repair costs was analyzed.
Secondly, the repair cost which was to e applied to the cubic functions change in terms of the values over time. Therefore, in order to ensure the monetary values of the currency over the analysis term, discount rates were applied. The discount rate to compensate for the differences in the values of the currency was set at 2.41%1).
1.3. Scope of Study
The scope of this study to analyze the settings and characteristics of the repair times was as follows; firstly, the target public rental houses for analysis were permeant rental houses, of which the summary were as shown in Table 1. The number of households was 1,563, which were divided into 12 pavilions. Secondly, the targets of analysis were separated into the interior spaces of buildings and the exterior spaces. Here, the lightings, wiring, installation of the kitchen equipment, wall paper, floor mats, replacement of the elevators, painting, water taps, unit household boilers, water-proofing of the roof top, water meter to measure the amount of water/hot water supplied, replacement of the telephone service outlets, amp-speakers for public announcements, and replacement of the sidewalk blocks, totalling to 13 components. These are mostly the required replacements for the interior spaces of a house. For the exterior spaces, roof-top water proofing and replacement of the sidewalk blocks are included. Thirdly, the houses were located in Seoul area, and the repair records of the public rental hoses were collected up to 2009. The contents of the survey included the time of repair works, the nature of the works, the number of areas that were repaired, and the unit prices or other repair work costs.
2. Method of determining the economic repair time
After completion of a building, it is necessary to establish maintenance strategies to cover various aspects of the building, such as the repair method, materials, repair plans, and the maintenance costs, so that the functions or performances of the buildings can be maintained above a certain level. in this case, the maintenance strategy can be established for the aspect of prevention and breakdown maintenances. In mose of the sites in practice, it is the breakdown maintenance method that is most widely used. While the importance of the preventive maintenance is somewhat underestimated. The maintenance strategy of the preventive method amy allow anticipation and preparation for the repair times, scope of repair works, contents of the repair works, amount of repair costs and how to secure such funds, which allows addressing all situations appropriately, as an advantage.
The repair time for the lightings, wiring, or other such components may be set in consideration of the repair costs and the level of wear and tear, In this case, the repair time in terms of the repair cost can be explained as the economic repair time. For this, the time when the accumulated cost becomes the highest in accordance with the age of the building or when there is a change in terms of the costs. On the other hand, the repair time by the level of wear and tear is intended to maintain the performance and function of the building over a certain level. This can be explained by the level of progress in terms of the wear and tears .
The economic aspect and the wear and tear aspect of the repair time is highly dependent on the record of the building, in which all the past repair works are recorded. This shows the mode of repair works, the costs and materials used in the maintenance works. And, it is assumed that the repair works in the future would be peformed in the same manner with those in the past.
2.1. The function between the age and the repair costs
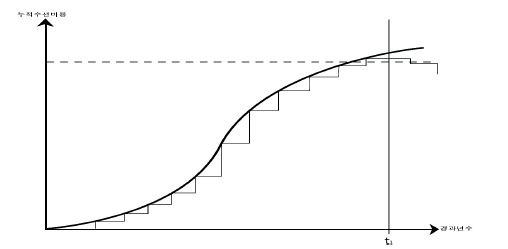
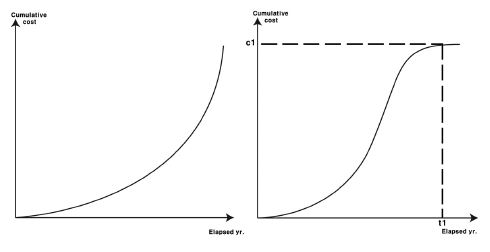
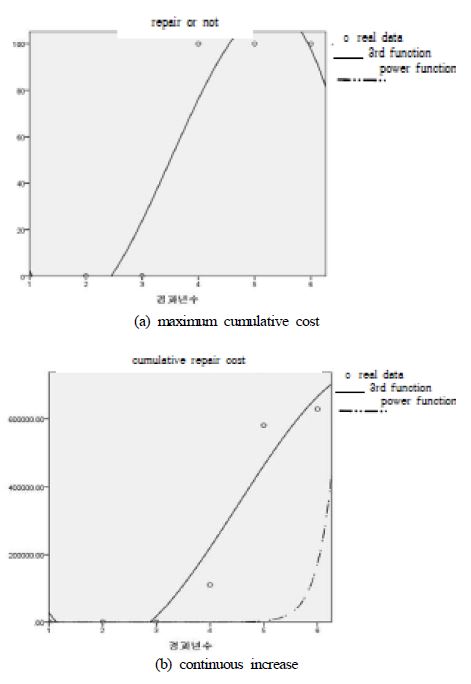
A building has to go through repair works of its components from the moment it is completed. At time, the relationship between the age in years and the cumulative repair cost are show in Fig.1 herein, which ca be calculated using a cubic function. Using this function, it is possible to calculated the time when the cumulative repair cost for the age in years is the highest. At this point, the cumulative repair cost can be explained in two difference cases where, respectively, the cumulative repair cost simply increases or the time when the maximum cumulative repair cost occurs is shown.
The type where the cost simply increases shows a patter In which the cumulative repair cost increases continuously over the year after completion of the building. Therefore, the appropriate repair time can be set by the increase or decrease ratio of the cumulative repair cost compared to the changes over time. That is, as the age of the building changes, the point where the changes of the cumulative repair cost is drastic can be suggested as the economic repair time. On the other hand, the point where the changes in the cumulative repair cost is not significant or where the maximum amount of the cumulative repair cost occurs, the repair cost stays rather flat from a certain point, instead of being spent on a continuous basis. In these two types of the patterns of the cumulative repair costs, it is more effective have shorter intervals between repair works when a principle of early, repetitive repairs in consideration nature of repair works is applied. (See Fig. 2)
The maintenance such as the repair of the parts or replacements require repair costs. In this case, it is more desirable that the repair works are performed when the repair cost’s increase is slowed or even decreasing rather then spending such costs immediately in response to wear and tear of the components after completion. That is, it is more desirable to find the optimal repair time by accumulating the repair costs assuming that it is spend continuously over time.
2.2. The model to calculate the economic repair time
After the completion of the building, the economic repair works in terms of remodelling or repair works can be divided into two categories, which are, the repetitive repair works in short intervals and performing one large scale repair work to cover them all at once. In this case, the economic repair time can be set using a probabilistic approach or a deterministic approach. The difference between these two is that it has rather continuous characteristics over time after completion rather than a discrete characteristic that occurs at a single point of time. Therefore, in a probabilistic approach, the economic repair time can be showed as a range rather than a single point of time. On the other hand, the deterministic method, with an assumption that it may become discrete over time, presents a single repair time.
In the probabilistic aspect and the deterministic aspect, the characteristics of the repair time can be used, so that the time of economic repair works can be approached in two major approaches. One of them is to make an estimation model using multi-term function to show the relationship between the number of years after completion and the cumulative repair cost. The other is to identify the time of repair costs when the cost increases continuously and simply in response to the number of years (the left figure of Fig.2)
In case of the former the cumulative cost can mark the time of the optimal repair time, where the cumulative cost against the number of years after completion reaches the peak. The peak of cumulative cost can be calculated using an estimation model of a multi-term function. In this study, a cubic function was used to identify the relationship between the cumulative cost and the number of years. Equation (1), which is a cubic function, was used to calculate the cumulative repair cost. 2).
| (1)3) |
In the case of the latter, early, repetitive repair works are desirable as a pattern of continued, simple increase appears. In this case, the time of the early repair should be set for preventive repair works.
By utilizing information such as above, the economic repair time can be explained in the following process. Firstly, the model to explain the time of repair and the cumulative repair cost was develop using a cubic function. In this case, the time when the maximum cumulative repair cost was reached was set as the time for the economic repair point. This is based on the assumption that the history of the repair works in the past maintains its tendencies in the future as well. Also, after the cumulative cost reaches its peak, the increase rate of it is reduced. Therefore, the cost looks lower than what it was during the previous time. Secondly, in the relationship between the time of repair and the cumulative repair cost, if the cost simply increases over time, the economic time of the repair works can be separated into the time when the increase rate of the cumulative cost compared to the time-change rate (Δcost/Δyear) is change drastically and where such a change show a smooth growth. It is more effective to use the early, repetitive repair works for the economic repair time in case of simple increases4). In the case where the change rate of the cumulative repair cost over the time-change rate(Δcost/Δyr) increases drastically, such a point of drastic increase can be set as the economic time of repair. This is because, after the time of rapid increase of cumulative repair costs, a drastic increase of the cumulative costs is obtained over time.
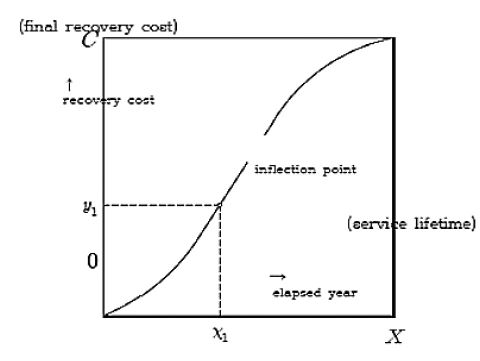
The time when there is a drastic change with the cumulative repair cost can be explained as the shifting point, which may be shown as in Fig. 4. A shifting point is where the rate of increase for the cumulative cost is changed5). The relationship between the cumulative cost and the time of repair can be shown as in a multi-term model such as Equation 1.
As shown in the Equation 1, a cubic function was used to identify the economic repair time from the relationship between the years after completion and the cumulative repair cost. The result of this analysis was as shown in Fig. 5 below. Firstly, depending on the number o f years, the cumulative cost increased continuously to flat out at a certain point. This is can be shown as in the upper pane in Fig. 5 ((a) where the max. cumulative cost is incurred.) Another is that the cumulative repair cost increase continuously over time, meaning simple increases. This can be shown as in the lower pane in Fig. 5 ((b) simple increases.)
3. The result of the analysis on the economic repair time
In this study, a cubic function was used to calculate the economic repair time f the interior space of a public rental multi-unit houses. With regard to the lightings, wiring, or other components, totalling to 11, were analyzed to estimate the parameters to set the economic repair time. The result of the estimation was as shown in Table 2, according to which the statistical values that explain the soundness of the cubic function model to explain the economic repair time are relatively appropriate. The determinant factor (R2) appears to be formed within a range of 0.6~0.9.
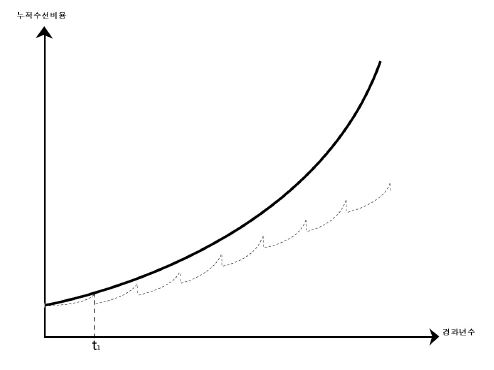
With regard to the 11 components, such as the lighting, wiring, kitchen equipment, wall paper, floor mats, replacement of an elevator, repainting, and water faucets, etc., Fig 6 shows a diagram of the relationship between the years in service and the cumulative costs. According to Fig. 6, the years in service was used as the independent variable, while the cumulative cost was used as the dependent variable. In this function, a tendency is shown that the cumulative cost reaches its peak at around the fourth year in service.
After the year when the cumulative cost reaches its peak, there appears a tendency of continued increase. That is, when the cumulative repair cost reaches is peak, there shifting point where the growth turns to decrease, or vice versa, is reached. After that, the value keeps increasing again.
Table 2 shows the economic repair time for the components of the interior spaces of a public rental house. According to this, the repair time comes in every 3.5 ~ 4.3 years. Since there is a tendency that the repair cost increase continuously after that, it appears that the pattern of early, repetitive repair work is more economical.
4. Conclusion and suggestions for future studies
A public rental house is occupied by one household after another as their designated terms of lease end. And, public authorities assumes the responsibility of maintenance of the facilities. After the completion of such buildings, repair costs are included in order for repair works or other maintenance activities. Here, it is important that the interval of repair works are set in consideration of the economy, such as the cost of repair works. For this, the relationship between the years in service and the cumulative cost of repair was determined for 11 key components. The results of this study were as follows;
Firstly, a cubic function was used as the multi-term equation to determine the economic repair time of the 11 key components or materials. The result f the analysis showed a shifting point, at which the cumulated repair cost reaches its peak after completion and then stars to increase again. Such a result appears to indicate that the repair pattern after the shifting point is a proportional, continuous increase. So repetitive, early repair works appears to be appropriate.
Secondly, the repair time of the key 11 components fall at some time between 3.5 years and 4.3 years. This implies that the at the fourth year or so, it is economical to do the repair jobs.
The repair time approach suggested in this study is based on the cost aspects of the repair works. When the cumulative cost reaches its peak, the timing of the early, repetitive repair works does not match with that of the actual timing of repair works. There is a certain limit for applying such a time to the actual maintenance. Therefore, determination of the maintenance schedule should consider the wear and tear, the method of works peformed, and other issues in a comprehensive manner. In addition, the service lives should be set in accordance with the performances and applicability of the components or materials, and the repair time should be set in consideration of the performances, costs, and the scope of repair.
Acknowledgments
“This paper is a result of 'Improvement of Building Maintenance System in Korea' which is a project of 2014 Basic Research Program of KICT”.
Notes
References
-
고은형, 외 2인 , (1998), “공동주택의 최적 경제수명 추정분석에 관한 연구”, 대한건축학회논문집 계획계, 14(4), p247-254.
Kang, H., Seo, J., (2003), “A Study on the Factor of Repair and Maintenance Costs of Public Rental Housing”, AIK Journal in Structural Area, 19(1), p145-152. -
이태경, 이강희, (2012), “공공 임대주택 마감자재 경제적 수선주기 추정”, 한국생태환경건축학회 학술발표대회논문집, 23, p75-81.
Lee, T., and Lee, K., (2012), “A Study on the Establishment of Economic Repair Cycle of the Public Rental Housing”, Proceedings of the Korea Institute of the Ecological Architecture and Environment, 23, p75-81. -
이강희, 채창우, (2006), "공동주택 구성재의 경제적 수선형태 설정 연구", 한국주거학회논문집, 7(5), p87-95.
Lee, K., Chae, C., (2006), “A Study on the Economic Repair Pattern of the Building Components in the Apartment Housing”, J. of Housing, 7(5), p87-95. -
장정희, 이강희, 임석호, (2006), “임대주택 부품의 수선위계 설정 제안에 관한 연구”, 대한건축학회논문집 계획계, 22(4), p31-38.
Jang, J., Lee, K., Lim, S., (2006), “A Study on Repair Hierarchy Proposal on the Rental Housing Components”, AIK Journal in Planning Area, 22(4), p31-38. - Ytchka, (1983), Building Maintenance, Kasima publication, p127-135.
- Robert, Johnson, (1990), The Economics of Building, John & Sons, p32-35.