Extension of Typical Meteorological Data and Energy Demand Analysis for Building Energy Efficiency Rating Certification System
ⓒCopyright Korea Institute of Ecological Architecture and Environment
Abstract
Meteorological data is one of the important factors in the calculation of building energy demand. The purposes of this study are to review the limitations of the typical meteorological data of ECO2 program and to create the new typical meteorological data and then analyze the building energy demands for additional regions which are not included in the existing 13 region in the ECO2 program. The extended typical meteorological data to a total of 33 regions were based on IWEC(International Weather for Energy Calculations) data files and were created in the form applicable to the building energy efficiency rating certification system. As a result of comparing the heating energy demands of a representative region with the surrounding regions in each of five regions in Korea, the variance of Cv(RMSE) ranged from 36% to 344% and MBE ranged from -32% to 190% for the whole regions. This suggests that the difference of heating energy demand may vary greatly depending on the region where the meteorological data is used and the meteorological data of more detailed regions is needed for reliable calculation of building energy demand
Keywords:
Typical Meteorological data, Builidng Energy efficiency rating, certification, ISO 13790, Building Energy Assessment키워드:
표준기상데이터, 건축물에너지효율등급 인증, 직산분리, ISO 13790, 건축물에너지 평가1. Introduction
Meteorological data in building energy simulations is a source of information on annual climate conditions. The 'typical meteorological data' used in the simulation is that of a 'typical year', which is made through statistical processing of the meteorological data for 15 to 30 years [1] .
In Korea, the building energy efficiency rating certification system is implemented [2], and ECO2 is used as a certification program. ECO2 simulation uses the typical meteorological data for 13 major regions in Korea [3]. However, these meteorological data do not reflect the meteorological information of the whole country, and when evaluating the energy efficiency rating of any region other than the 13 major regions, it may be ambiguous which meteorological data should be used.
In the current building energy efficiency rating system, the meteorological data of the nearest surrounding region is used instead when evaluating the energy efficiency rating of any region other than the 13 major regions[4]. For example, Suwon uses the meteorological data of Seoul, but it is also adjacent to Incheon. Pohang adjacent to the coast, which is the closest distance to Daegu, is likely to have problems in using the meteorological data of Daegu which is characteristic of inland climate.
In this study, the limitations of ECO2 typical meteorological data used for the building energy efficiency rating certification are examined and the typical meteorological data of the additional regions not included in the existing 13 major regions are created. Based on the created typical meteorological data, the heating energy demands of the additional regions are also analyzed.
2. Necessity to expand the existing typical meteorological data
In relation to the building energy efficiency rating certification, the necessity to expand the existing typical meteorological data has been mentioned by Hideki Kikumoto et al. [5] and Lee Sung Jin et al. [6]. The operational regulations of the building energy efficiency rating certification system provide only the meteorological data of 13 major regions for energy simulation [3], and thereby there is a limit to obtain the simulation results for any region other than those major regions. Therefore, it is necessary to acquire the meteorological data of additional regions in order to reflect the local characteristics of meteorological data and to improve the reliability of simulation results in building energy evaluation,
The current operational regulations, if any region has no meteorological data, recommend use of the typical meteorological data of the surrounding regions . For example, Pohang uses the meteorological data of Daegu or Busan, and Yeosu uses the meteorological data of the neighboring Gwangju or Mokpo. However, this may have a large influence on energy demand calculation results as well as the meteorological data itself depending on which regions to select.
To verify this problem, the present study takes the cases of Pohang and Yeosu as an example. The energy demands are calculated when the meteorological data of the surrounding major regions (Daegu and Busan in case of Pohang and Gwangju and Mokpo in case of Yeosu) are used, and the difference between the two calculation results are compared. In this calculation, the reference model house and the calculation procedure are based those described in Chapter 4.
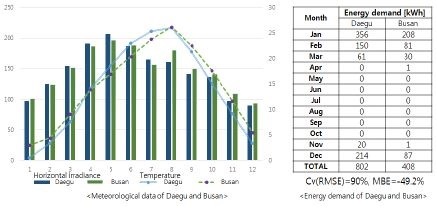
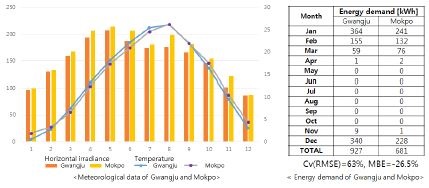
Fig. 1 shows the meteorological data and energy demand calculation results of Daegu and Busan, and Fig. 2 shows those of Gwangju and Mokpo.
The statistics, Cv(RMSE)(Coefficient of Variation of the Root Mean Squared Error) and MBE(Mean Bias Error), are defined as follows [7].
| (1) |
| (2) |
| (3) |
| (4) |
M = simulation result value (kWh)
S = another simulation result value (kWh)
Ninterval = the number of time intervals
These statistics, which are commonly used to verify the measured value of building energy and the calculated value of model simulation, are used in this study to compare the deviation between simulation results according to different meteorological data. In the case of Pohang, as shown in the figure, it showed a difference of Cv (RMSE) 90%, MBE -49.2% in the heating energy demand of Daegu and Busan, and in the case of Yeosu, it showed a difference of Cv (RMSE) 63% and MBE -26.5% in the heating energy demand Gwangju and Mokpo. This suggests: If there are no typical meteorological data for any region to be evaluated, the use of the meteorological data of the neighboring 13 regions does not reflect the meteorological characteristics of the corresponding region, and thus it may have a large impact on calculation results.
3. Creation of IWEC-based extended typical meteorological data
IWEC (International Weather for Energy Calculations) is the representative meteorological data for building energy calculation which is provided by the American Society of Heating, Refrigerating and Air Conditioning Engineers (ASHRAE) [8]. It was made with the data of National Climatic Data Center (NC) in the USA. For Korea, the IWEC provides 69 kinds of meteorological data including temperature, humidity, solar radiation, wind speed, cloudiness and illumination for 33 regions: Seoul, Busan, Incheon, Daegu, Daejeon, Gangreung, Gwangju, Wonju, Chuncheon, Jeonju, Cheongju, Mokpo, Jeju, Andong, Cheorwon, Chupwolryeong, Daegwallyeong, Gunsan, Jinju, Masan, Pohang, Seogwipo, Seosan, Sokcho, Suwon, Tongyeong, Uljin, Ulleungdo, Ulsan, Wando, Yeongwol , Yeosu, and Donghae) [9].
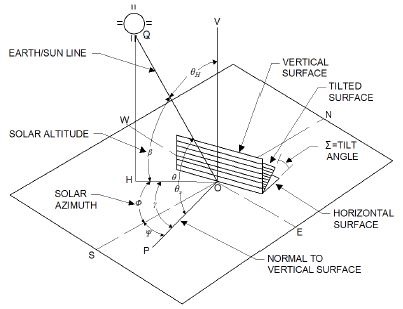
However, the IWEC meteorological data can’t be directly applied to the simulation of building energy efficiency rating certification. To calculate the energy demand, 8,760 hourly raw data should be converted into monthly data, and calculations for solar altitude and total solar irradiance by azimuth are also needed. The solar altitude and azimuth separation are calculated by the method of ASHRAE Fundamental 2013: Calculating clear-sky solar radiation & Transposition to receiving surfaces of various orientations [10].
Fig. 3 shows the solar angles to the vertical and horizontal planes. The solar altitude was calculated using the following equations (5) - (7).
| (5) |
| (6) |
| (7) |
where
β = Solar altitude (°)
L = Latitude(°)
n = Day of year
AST = Apparent solar time
The total solar irradiance of horizontal surface, (Et,h), and the total solar irradiance by directions, (Et,v) are obtained using the solar altitude calculated from the above equations and the following equations (8) to (14).
| (8) |
| (9) |
| (10) |
| (11) |
| (12) |
| (13) |
| (14) |
where
Et,h = Horizontal surface total solar irradiance (W/m2)
Et,v = Vertical surface total solar irradiance (W/m2)
Ed = Diffuse solar irradiance (W/m2)
Eb,h = Horizontal surface direct normal irradiance (W/m2)
Eb,v = Vertical surface direct normal irradiance (W/m2)
Eb = Direct normal irradiance (Solar radiation received in a collimated beam on a surface normal to the sun (W/m2)
Φ = Solar azimuth (°)
Ψ = Surface azimuth (°)
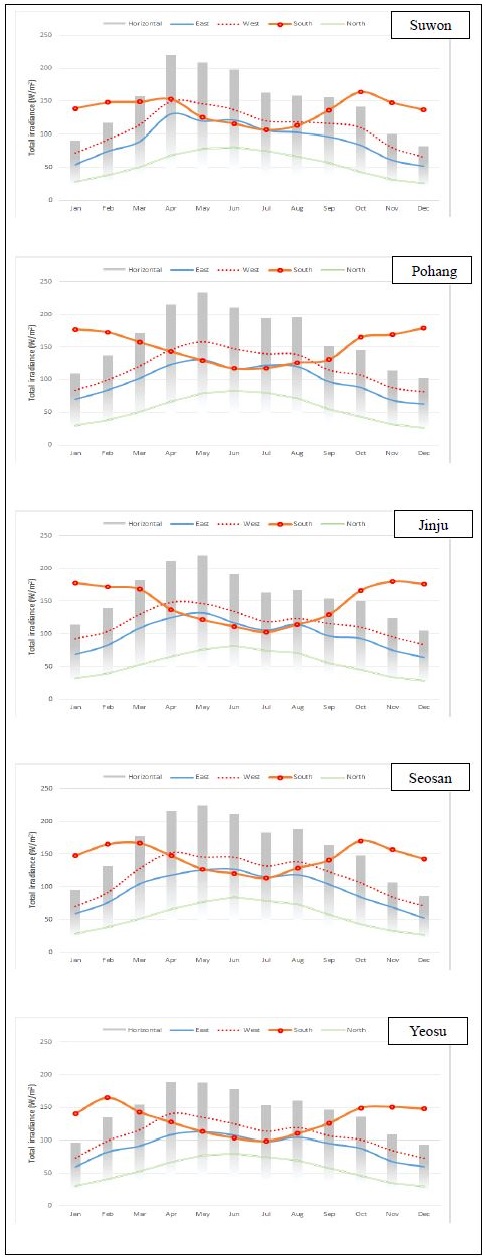
Table I shows the temperature and total solar irradiance of 33 regions in Korea provided by IWEC. With these data, the total solar irradiances of horizontal surface and the total solar irradiance by directions were calculated, which showed different values depending on different regions but a similar pattern in the monthly distribution. Fig. 4, as an example of those calculation results, shows the total solar irradiance of horizontal surface and total solar irradiance by the directions of east, west, north, and south for additional representative regions (Suwon, Pohang, Seosan, Yeosu, and Jinju) which are not included in the 13 major regions of ECO2 program and also were selected one by one from the 5 region groups of the whole country.
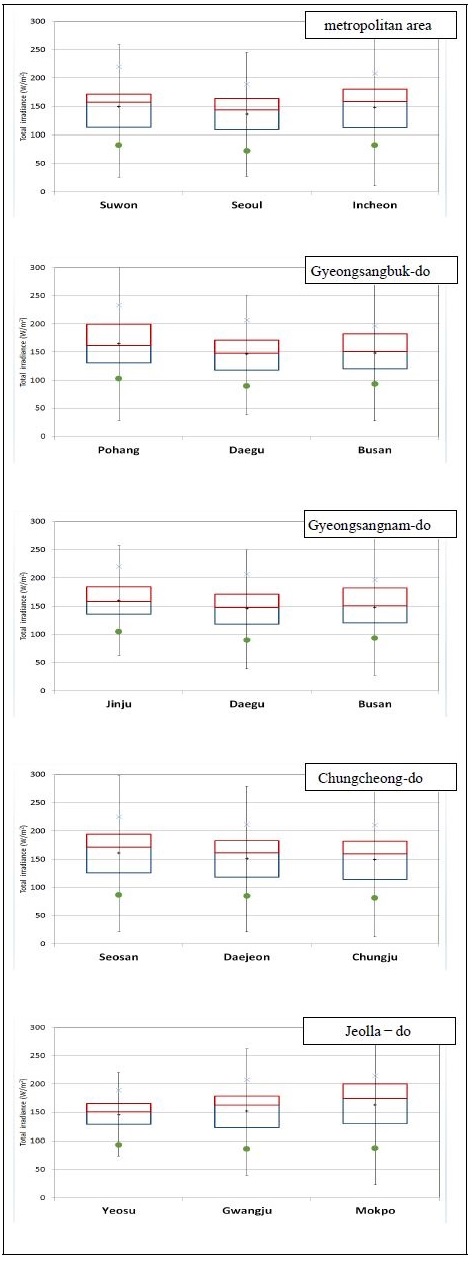
Fig. 5 is shown in the form of skeletal box-and-whishker plot of the total irradiance of horizontal surface for the additionally selected representative regions and the existing regions around them (Seoul and Incheon in the case of Suwon, Daegu and Busan in case of Pohang, in case of Jinju Daegu and Busan in case of Jinju, Daejeon and Cheongju in case of Seosan, Gwangju and Mokpo in case of Yeosu, which are provided with the meteorological data of ECO2). As shown in the figure, the averaged total solar irradiance of horizontal surface was 149.19 W/m2, 152.63 W/m2, 146.27 W/m2 in the case of Suwon-Seoul-Incheon, respectively; 164.84 W/m2, 158.40 W/m2, 165.48 W/m2 in the case of Pohang-Daegu-Busan, respectively; 167.34 W/m2, 158.40 W/m2, 165.48 W/m2 in the case of Jinju-Daegu-Busan, respectively; 161.12 W/m2, 161.55 W/m2, 162.47 W/m2 in the case of Seosan-Daejeon-Cheongju, respectively, and 144.92 W/m2, 162.97 W/m2, 165.95 W/m2 in the case of Yeosu-Gwangju–Mokpo, respectively. As the Cv(RMSE) deviation of the averaged total solar irradiance of horizontal surface between a representative region in the five region groups and the surrounding regions, Suwon showed 6% and 8% for Seoul and Incheon, respectively; Pohang showed 7% and 5% for Daegu and Busan, respectively; Jinju showed 6% and 9% for Daegu and Busan, respectively; Seosan showed 4% and 7% for Daejeon and Cheongju, respectively, and Yeosu showed 14% and 17% for Gwangju and Mokpo, respectively. The overall deviation of Cv(RMSE) ranged from minimum 4% to maximum 17%.
4. Energy demand analysis using typical meteorological data
4.1. Calculation method of building energy demand
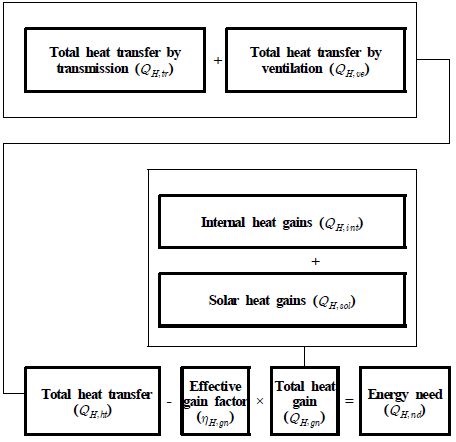
Since ECO2 simulation cannot directly calculate the building energy demands using the extended meteorological data[11], this study uses ISO 13790: Monthly Calculation Method[12]. Only the heating energy demand is considered in the calculations. The calculation process of the heating energy demands is shown in Fig. 6. The heating energy demand in this calculation method is expressed by the following equation:
| (15) |
where
QH,nd = Heating energy demand (MJ)
QH,ht = Total heat transfer in heating mode (MJ)
ηH,gn = Effective gain factor in heating mode
QH,gn = Total heat gain in heating mode (MJ)
In the equation (15), the total heat transfer (QH,ht) in the heating mode refers to the heat transfer by transmission and ventilation, and the total heat gain (QH,gn) in the heating mode refers to the internal heat gains and solar heat gains. The effective gain factor (ηH,gn) is a coefficient indicating the degree to which the amount of heat obtained in the heating mode is utilized to reduce the amount of heat loss, and it is calculated by reflecting the heat-balance ratio and the heat capacity of structure.
The total heat transfer (QH,ht) and the total heat gain (QH,gn) in the heating mode are as written in equations (16) and (17), and the total heat transfer (QH,ht) is determined by the heating set-point temperature (θint,set) and the monthly averaged outside temperature (θe)
| (16) |
| (17) |
| (18) |
| (19) |
where
QH,tr = Heat transfer by transmission in heating mode (MJ)
QH,ve = Heat transfer by ventilation in heating mode (MJ)
Htr = Heat transfer coefficient by transmission (W/K)
Hve = Heat transfer coefficient by ventilation (W/K)
θint,set = Set-point temperature (℃)
t = period of time (Mega seconds)
The effective gain factor (ηH,gn) in the equation (15) for calculating the heating energy demand is calculated by the following equations (20) to (26).
| (20) |
| (21) |
| (22) |
| (23) |
| (24) |
| (25) |
| (26) |
where
γH : Heat-balance Ratio in heating mode
aH : Numerical parameter dependimg on the time constant(τH)
aH,0 : Reference numerical parameter
τ : Time constant of the building zone (h)
τH,0 : Reference time constant (h)
Cm : The sum of heat capacity of the building (J/K)
κj : The heat capacityof factor (J/ (m2K))
Aj : Area of building factor j (m2)
The reference numerical parameter (aH,0) and the reference time constant (τH,0) in the equation (24) are presented in the table of ISO 13790. Each country uses its own values according to the situation, but if there are no available values, the values of Table. 2 are used [12].
4.2. Reference model
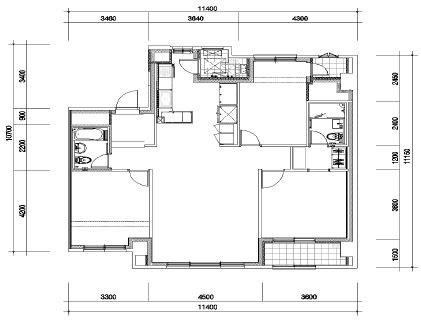
A unit area plane of the reference model house has a specific area of 85㎡ (Fig. 7). The extended plane on 3Bay was selected as the plane configuration of the evaluation model, and the input conditions [4] for the calculation of the energy demand are shown in Table. 3.
4.3. Energy demand analysis and application of the extended typical meteorological data
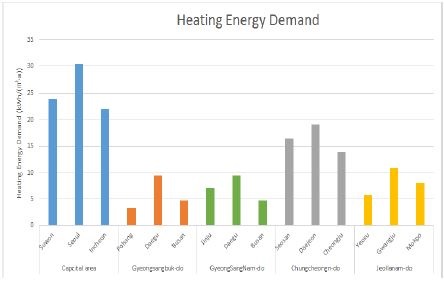
The annual heating energy demand per unit area calculated for the reference model is shown in Fig. 8. The annual heating energy demand per unit area of Suwon, Seoul, and Incheon located in the metropolitan area was 24.01 kWh/(m2·a), 30.32 kWh/(m2·a), 22.00 kWh/(m2·a), respectively, while the Cv(RMSE) of Seoul and Incheon based on Suwon was 49% and 36%, respectively, and MBE showed the difference of 26% and –8%, respectively. In Gyeongsangbuk-do, the heating energy demand of Pohang, Daegu and Busan was 3.26 kWh/(m2·a), 9.45 kWh/(m2·a), 4.80 kWh/(m2·a), and the Cv (RMSE) of Daegu and Busan based on Pohang was 344% and 84%, respectively, and the MBE was 190% and 47%. In Gyeongsangnam-do, the heating energy demand of Jinju, Daegu and Busan was 7.03 kWh/(m2·a), 9.45 kWh/(m2·a), 4.80 kWh/(m2·a) and the Cv (RMSE) of Daegu and Busan based on Jinju was 61%, and 69%, and the MBE was 34% and -32%. In Chungcheong-do, the heating energy demand of Seosan, Daejeon and Cheongju was 16.43 kWh/(m2·a), 19.13 kWh/(m2·a), 13.93 kWh/(m2·a), and the Cv (RMSE) of Daejeon and Cheongju based on Seosan was 38%, 37%, and the MBE was 16% and -15%. Finally, in Jeolla–do, the heating energy demand of Yeosu, Gwangju and Mokpo was 5.76 kWh/(m2·a), 10.93 kWh/(m2·a), 8.03 kWh/(m2·a), respectively, and the Cv (RMSE) of Gwangju and Mokpo based on Yeosu was 196%, 130%, respectively, and the MBE showed a deviation of 90%, 39% respectively. The results are summarized in Table. 4.

Comparison of additional regions with the surrounding regions for heating energy demand and statistical analysis.
As a result of calculation, the heating energy demand of the additional region and the surrounding regions showed a great difference. In addition, the statistical analysis showed a variation of 36%~344% Cv (RMSE) and -32%~190% MBE, which indicates that the heating energy calculation result significantly varies depending on the meteorological data of the corresponding region.
5. Conclusion
This study newly created the typical meteorological data of 33 regions in Korea using the meteorological data provided by IWEC. With these typical meteorological data, the heating energy demands of an additional region and the surrounding ECO2 regions for the five region groups were also analyzed. As a result of the analysis, the heating energy demand of the additional region and the surrounding ECO2 regions varied by 36%~344% in Cv (RMSE) and –32%~190% in MBE. This suggests that the ECO2 typical meteorological data has a limit to apply to any region far from the existing 13 major regions and the typical meteorological data of more regions are needed in order to evaluate more reliable building energy demands. However, since the typical meteorological data cannot be expanded indefinitely, further study should be carried out to identify valid territory when evaluating the surrounding region using the meteorological data of a representative region and to quantify the number of typical meteorological data needed in Korea.
Acknowledgments
“This work was conducted under framework of the research and development program of the Korea Institute of Energy Research(B7-9302-01) and (B6-2462)”
References
- Seo, Dong Hyun, "Standard meteorological data of domestic and international meteorological data and status and utilization of standard building model", The Korean Society for Environmental Ecology Facility Journal, (2015, Jul).
- Kim, Ji-Yeon, "Overview and Status of Energy Efficiency Rating Certification System for Buildings", Korea Institute of Machinery and Materials Equipment Journal, (2010, Oct).
- (Chun, Jung-Gil, Evaluation of Building Energy Efficiency Rating of the Business Buildings : A Case Study, Conference Jounal of The Society of Air-Conditionong And Refrigerating Engineers of Korea, (2011, Jul).
- Korea Energy Corporation, "Operational regulations on Energy Efficiency Rating for Buildings", (2013, Sep).
- Hideki, kikumoto, Study on the future weather data considering the global and localclimate change for building energy simulation, Jounal of Sustainable Cities and Society, (2014).
- Lee, Sung Jin, Analysis and Extendability Review of Typical Weather Data for Building Energy Efficiency Rating System, Conference Jounal of The Korean Solar Energy Society, (2016, Oct).
- U.S. DEPARTMENT OF ENERGY, M&V Guidelines: Measurement and Verification for Federal Energy Projects version 4.0, (2015).
- ASHRAE, ASHRAE International Weatehr Files For Energy Calculations 2.0 (IWEC2), https://www.ashrae.org/ (2016).
- ASHRAE, IWEC2 User Manual and Final Report, (2011, Oct).
- ASHRAE, ASHRAE Fundamental Chapter 14 Climate Design Information, ASHRAE Handbook, (2013).
- Jeon, Jiwoon, A study on the energy-saving design for public office buildings using ECO2 program, Jungang Univ thesis, (2013, Aug).